Analogy in variable space
Now let's use a vehicle as an analogy. A few years ago
Chrysler introduced a model called Intrepid. In this model the four
wheels are spread out so that the car is better-supported and thus
stable. We can look at a regression model as an automobile model--it
requires support and stability. Now let us examine the following
regression model.
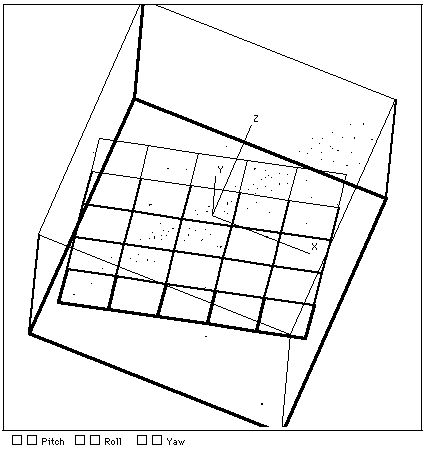
The following figure is a 3D plot of three
hypothetical variables--X, Y, and Z, with a regression plane. The
regression plane is a function defined by the data points.
Geometrically, we can think of the plane as an object that is supported
by the points, just like a car is supported by four wheels. There
exists the threat of collinearity between Y and Z if the dots do not
spread out in the X space enough to provide stable support for the
plane. If the points are too close to each other, the model would
collapse.
If the regression plane is too abstract to you, let's
look at some physical objects. In the following pictures, the paper is
the regression plane and the fingers are the data points. In the left
picture my fingers spread out and thus the plane is well-supported. On
the contrary, in the right picture my fingers are too close to each
other. As a result, the plane tends to fall down.
Navigation
Index
Simplified Navigation
Table of Contents
Search Engine
Contact